Study on the efficiency and temperature robustness of chirped PPLN crystal in 1064nm frequency doubling experiment - 02

2. Theoretical analysis

2.1 Temperature robustness

Temperature robustness refers to the stability of the frequency-doubled crystal with respect to temperature. Specifically, when the temperature fluctuates, the power of the frequency-doubled light will not be greatly affected. The influence of temperature on the frequency doubling process mainly comes from the influence on the phase mismatch. During the frequency doubling process, in the case of small signal approximation, the power of frequency doubled light can be expressed as:

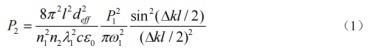
In equation 1, l is the length of the frequency doubling crystal; d_eff is the effective nonlinear coefficient; c is the speed of light in vacuum; ε₀ is the vacuum dielectric constant; P_i , λ_i, n_i , and ω_i are the power, wavelength, refractive index and beam waist radius of the fundamental frequency beam and frequency-doubled beam respectively. The subscripts i=1, 2 correspond to the fundamental frequency beam and the frequency-doubled beam respectively; the phase mismatch amount Δk = k₂ -2k₁, in which wave vector k_i=(n_i2π)/λ_i. It can be seen from equation 1 that only when the phase matching condition is met, that is, when Δk=0, the maximum frequency-doubled optical power can be obtained. In the process of quasi-phase matching, since the spontaneous polarization direction of the nonlinear crystal is artificially and periodically changed, the polarization period Λ will introduce the inverse lattice vector G_m. The relationship between the inverse lattice vector and the polarization period is: 

G_m=m(2π/Λ)    (2)

Where m represents the number m order quasi-phase matching. Then the phase mismatch in the frequency doubling process can be rewritten as:

Δk' = k₂ -2k₁-G_m      (3)

It is known from the Sellmeier equation: when the temperature changes, the refractive index n_i will also be affected, which in turn affects the phase mismatch Δk. Since the polarization period of PPLN (www.wisoptic.com) is a single period and the introduced reciprocal vector is a fixed value, phase matching Δk' = 0 can only be achieved under specific wavelengths and specific temperature conditions. Therefore we can know that the key point to achieve temperature robustness is to expand the gain bandwidth of the polarized crystal so that the inverse lattice vector can cover a certain range and the phase mismatch can still satisfy Δk' = 0 when the temperature changes. In other words, the temperature robustness is linked to the gain bandwidth of the crystal. The larger the gain bandwidth, the better the temperature robustness of the crystal. The polarization period of PPLN can be expressed as a function of position as follows:

In the formula, D_g is the chirp degree of CPPLN crystal (www.wisoptic.com) , and Λ₀ is the initial period of CPPLN. It can be seen from Equation 4 that the polarization period of CPPLN covers Λ₁ = 2πΛ₀ and Λ₂ = Λ₀/[(1+D_gΛ₀l)/2π]. And it changes continuously within this range, that is, the CPPLN of this structure can provide an inverse lattice vector that changes continuously within a certain range. When the phase mismatch k changes within this range due to temperature, the inverse lattice vector of CPPLN will perfectly compensate for its changing value to ensure Δk' = 0, that is, the conversion efficiency of frequency-doubled light can be maintained at a very high level within a certain temperature range. However, it should be noted that the larger the polarization period range of CPPLN is not the better, because the increase in chirp will lead to the reduction of the effective nonlinear coefficient. Therefore, in the actual production process, a balance between temperature robustness and the highest frequency multiplication efficiency must be made.